| << Chapter < Page | Chapter >> Page > |
It follows from [link] that we can parameterize all cylinders of the form If S is a cylinder given by equation then a parameterization of S is
We can also find different types of surfaces given their parameterization, or we can find a parameterization when we are given a surface.
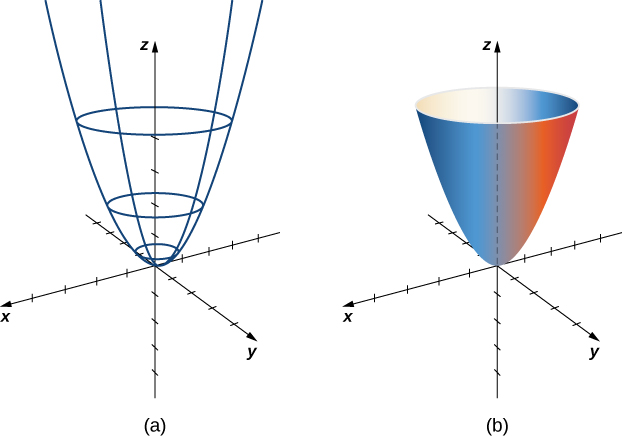
Describe surface S parameterized by
Notice that if u is held constant, then the resulting curve is a circle of radius u in plane Therefore, as u increases, the radius of the resulting circle increases. If v is held constant, then the resulting curve is a vertical parabola. Therefore, we expect the surface to be an elliptic paraboloid. To confirm this, notice that
Therefore, the surface is elliptic paraboloid ( [link] ).
Give a parameterization of the cone lying on or above the plane
The horizontal cross-section of the cone at height is circle Therefore, a point on the cone at height u has coordinates for angle v . Hence, a parameterization of the cone is Since we are not interested in the entire cone, only the portion on or above plane the parameter domain is given by ( [link] ).
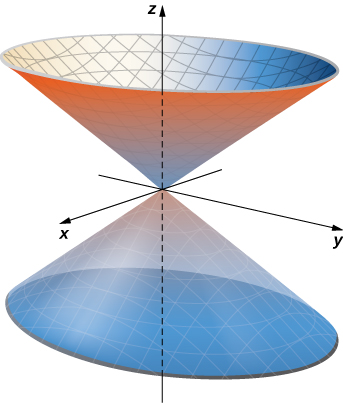
Give a parameterization for the portion of cone lying in the first octant.
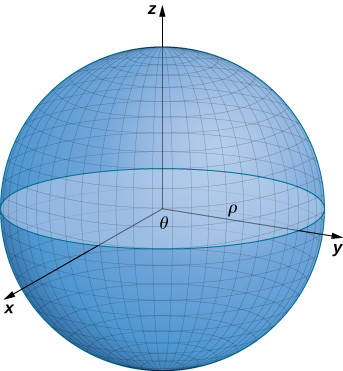
We have discussed parameterizations of various surfaces, but two important types of surfaces need a separate discussion: spheres and graphs of two-variable functions. To parameterize a sphere, it is easiest to use spherical coordinates. The sphere of radius centered at the origin is given by the parameterization
The idea of this parameterization is that as sweeps downward from the positive z -axis, a circle of radius is traced out by letting run from 0 to To see this, let be fixed. Then
This results in the desired circle ( [link] ).
Finally, to parameterize the graph of a two-variable function, we first let be a function of two variables. The simplest parameterization of the graph of is where x and y vary over the domain of ( [link] ). For example, the graph of can be parameterized by where the parameters x and y vary over the domain of If we only care about a piece of the graph of —say, the piece of the graph over rectangle —then we can restrict the parameter domain to give this piece of the surface:

Notification Switch
Would you like to follow the 'Calculus volume 3' conversation and receive update notifications?