0.1 Proofs, identities, and toolkit functions

<< Chapter < Page

Algebra and trigonometry Page 1 / 1

Chapter >> Page >

Appendix

Important proofs and derivations

Product Rule

$\log_{a} x y = \log_{a} x + \log_{a} y$

Proof:

Let $m = \log_{a} x$ and $n = \log_{a} y .$

Write in exponent form.

$x = a^{m}$ and $y = a^{n} .$

Multiply.

$x y = a^{m} a^{n} = a^{m + n}$

$\begin{matrix} a^{m + n} & = & x y \\ \log_{a} (x y) & = & m + n \\ = & \log_{a} x + \log_{b} y \end{matrix}$

Change of Base Rule

$\begin{array}{l} \log_{a} b = \frac{\log_{c} b}{\log_{c} a} \\ \log_{a} b = \frac{1}{\log_{b} a} \end{array}$

where $x$ and $y$ are positive, and $a > 0, a \neq 1.$

Proof:

Let $x = \log_{a} b .$

Write in exponent form.

$a^{x} = b$

Take the $\log_{c}$ of both sides.

$\begin{matrix} \log_{c} a^{x} & = & \log_{c} b \\ x \log_{c} a & = & \log_{c} b \\ x & = & \frac{\log_{c} b}{\log_{c} a} \\ \log_{a} b & = & \frac{\log_{c} b}{\log_{a} b} \end{matrix}$

When $c = b,$

$\log_{a} b = \frac{\log_{b} b}{\log_{b} a} = \frac{1}{\log_{b} a}$

Heron’s Formula

$A = \sqrt{s (s - a) (s - b) (s - c)}$

where $s = \frac{a + b + c}{2}$

Proof:

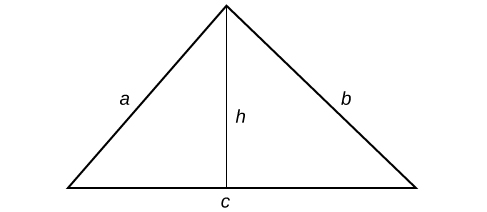
Let $a,$ $b,$ and $c$ be the sides of a triangle, and $h$ be the height.

A triangle with sides labeled: a, b and c. A line runs through the center of the triangle, bisecting the top angle; this line is labeled: h.

So $s = \frac{a + b + c}{2}$ .

We can further name the parts of the base in each triangle established by the height such that $p + q = c .$

A triangle with sides labeled: a, b, and c. A line runs through the center of the triangle bisecting the angle at the top; this line is labeled: h. The two new line segments on the base of the triangle are labeled: p and q.

Using the Pythagorean Theorem, $h^{2} + p^{2} = a^{2}$ and $h^{2} + q^{2} = b^{2} .$

Since $q = c - p,$ then $q^{2} = {(c - p)}^{2} .$ Expanding, we find that $q^{2} = c^{2} - 2 c p + p^{2} .$

We can then add $h^{2}$ to each side of the equation to get $h^{2} + q^{2} = h^{2} + c^{2} - 2 c p + p^{2} .$

Substitute this result into the equation $h^{2} + q^{2} = b^{2}$ yields $b^{2} = h^{2} + c^{2} - 2 c p + p^{2} .$

Then replacing $h^{2} + p^{2}$ with $a^{2}$ gives $b^{2} = a^{2} - 2 c p + c^{2} .$

Solve for $p$ to get

$p = \frac{a^{2} + b^{2} - c^{2}}{2 c}$

Since $h^{2} = a^{2} - p^{2},$ we get an expression in terms of $a,$ $b,$ and $c .$

$\begin{matrix} h^{2} & = & a^{2} - p^{2} \\ = & (a + p) (a - p) \\ = & [a + \frac{(a^{2} + c^{2} - b^{2})}{2 c}] [a - \frac{(a^{2} + c^{2} - b^{2})}{2 c}] \\ = & \frac{(2 a c + a^{2} + c^{2} - b^{2}) (2 a c - a^{2} - c^{2} + b^{2})}{4 c^{2}} \\ = & \frac{({(a + c)}^{2} - b^{2}) (b^{2} - {(a - c)}^{2})}{4 c^{2}} \\ = & \frac{(a + b + c) (a + c - b) (b + a - c) (b - a + c)}{4 c^{2}} \\ = & \frac{(a + b + c) (- a + b + c) (a - b + c) (a + b - c)}{4 c^{2}} \\ = & \frac{2 s \cdot (2 s - a) \cdot (2 s - b) (2 s - c)}{4 c^{2}} \end{matrix}$

Therefore,

$\begin{matrix} h^{2} & = & \frac{4 s (s - a) (s - b) (s - c)}{c^{2}} \\ h & = & \frac{2 \sqrt{s (s - a) (s - b) (s - c)}}{c} \end{matrix}$

And since $A = \frac{1}{2} c h,$ then

$\begin{matrix} A & = & \frac{1}{2} c \frac{2 \sqrt{s (s - a) (s - b) (s - c)}}{c} \\ = & \sqrt{s (s - a) (s - b) (s - c)} \end{matrix}$

Properties of the Dot Product

$u \cdot v = v \cdot u$

Proof:

$\begin{matrix} u \cdot v & = ⟨ u_{1}, u_{2}, ... u_{n} ⟩ \cdot ⟨ v_{1}, v_{2}, ... v_{n} ⟩ \\ = u_{1} v_{1} + u_{2} v_{2} + ... + u_{n} v_{n} \\ = v_{1} u_{1} + v_{2} u_{2} + ... + v_{n} v_{n} \\ = ⟨ v_{1}, v_{2}, ... v_{n} ⟩ \cdot ⟨ u_{1}, u_{2}, ... u_{n} ⟩ \\ = v \cdot u \end{matrix}$

$u \cdot (v + w) = u \cdot v + u \cdot w$

Proof:

$\begin{matrix} u \cdot (v + w) & = ⟨ u_{1}, u_{2}, ... u_{n} ⟩ \cdot (⟨ v_{1}, v_{2}, ... v_{n} ⟩ + ⟨ w_{1}, w_{2}, ... w_{n} ⟩) \\ = ⟨ u_{1}, u_{2}, ... u_{n} ⟩ \cdot ⟨ v_{1} + w_{1}, v_{2} + w_{2}, ... v_{n} + w_{n} ⟩ \\ = ⟨ u_{1} (v_{1} + w_{1}), u_{2} (v_{2} + w_{2}), ... u_{n} (v_{n} + w_{n}) ⟩ \\ = ⟨ u_{1} v_{1} + u_{1} w_{1}, u_{2} v_{2} + u_{2} w_{2}, ... u_{n} v_{n} + u_{n} w_{n} ⟩ \\ = ⟨ u_{1} v_{1}, u_{2} v_{2}, ..., u_{n} v_{n} ⟩ + ⟨ u_{1} w_{1}, u_{2} w_{2}, ..., u_{n} w_{n} ⟩ \\ = ⟨ u_{1}, u_{2}, ... u_{n} ⟩ \cdot ⟨ v_{1}, v_{2}, ... v_{n} ⟩ + ⟨ u_{1}, u_{2}, ... u_{n} ⟩ \cdot ⟨ w_{1}, w_{2}, ... w_{n} ⟩ \\ = u \cdot v + u \cdot w \end{matrix}$

$u \cdot u = {| u |}^{2}$

Proof:

$\begin{matrix} u \cdot u & = ⟨ u_{1}, u_{2}, ... u_{n} ⟩ \cdot ⟨ u_{1}, u_{2}, ... u_{n} ⟩ \\ = u_{1} u_{1} + u_{2} u_{2} + ... + u_{n} u_{n} \\ = u_{1}^{2} + u_{2}^{2} + ... + u_{n}^{2} \\ = | ⟨ u_{1}, u_{2}, ... u_{n} ⟩ |^{2} \\ = v \cdot u \end{matrix}$

Standard Form of the Ellipse centered at the Origin

$1 = \frac{x^{2}}{a^{2}} + \frac{y^{2}}{b^{2}}$

Derivation

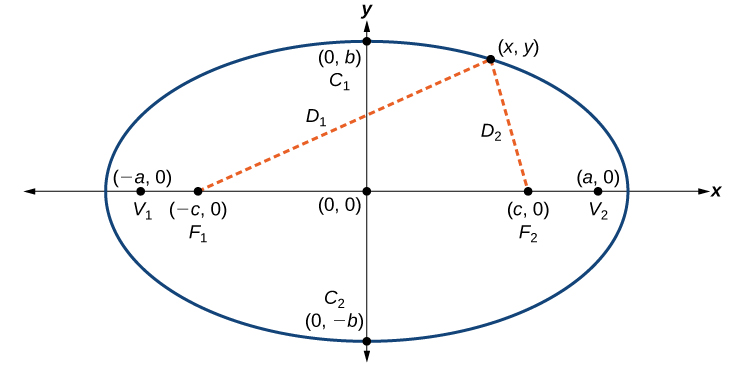
An ellipse consists of all the points for which the sum of distances from two foci is constant:

$\sqrt{{(x - (- c))}^{2} + {(y - 0)}^{2}} + \sqrt{{(x - c)}^{2} + {(y - 0)}^{2}} = constant$

An ellipse centered at the origin on an x, y-coordinate plane. Points C1 and C2 are plotted at the points (0, b) and (0, -b) respectively; these points appear on the ellipse. Points V1 and V2 are plotted at the points (-a, 0) and (a, 0) respectively; these points appear on the ellipse. Points F1 and F2 are plotted at the points (-c, 0) and (c, 0) respectively; these points appear on the x-axis, but not the ellipse. The point (x, y) appears on the ellipse in the first quadrant. Dotted lines extend from F1 and F2 to the point (x, y).

Consider a vertex.

An ellipse centered at the origin. The points C1 and C2 are plotted at the points (0, b) and (0, -b) respectively; these points are on the ellipse. The points V1 and V2 are plotted at the points (-a, 0) and (a, 0) respectively; these points are on the ellipse. The points F1 and F2 are plotted at the points (-c, 0) and (c, 0) respectively; these points are on the x-axis and not on the ellipse. A line extends from the point F1 to a point (x, y) which is at the point (a, 0). A line extends from the point F2 to the point (x, y) as well.

Then, $\sqrt{{(x - (- c))}^{2} + {(y - 0)}^{2}} + \sqrt{{(x - c)}^{2} + {(y - 0)}^{2}} = 2 a$

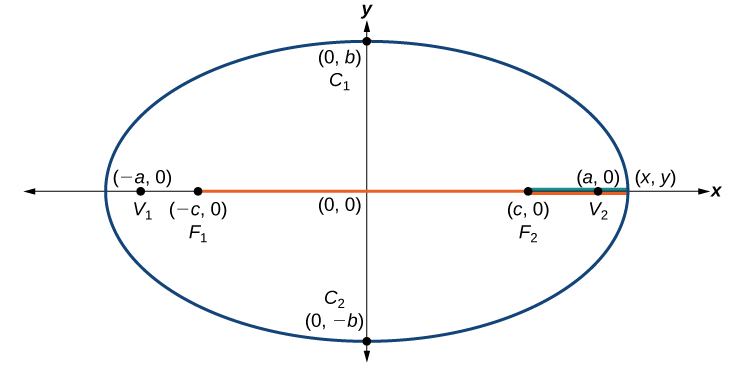
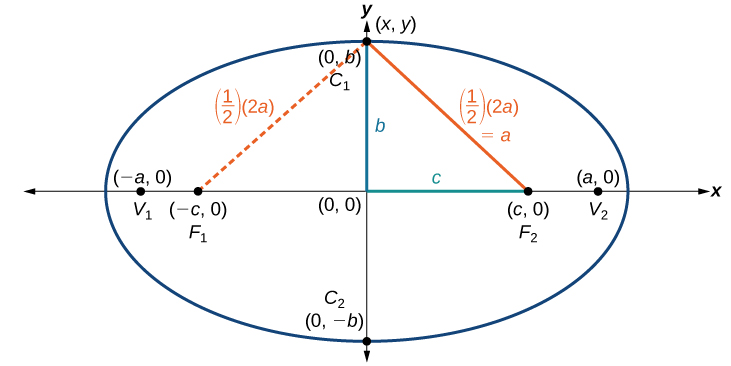
Consider a covertex.

Then $b^{2} + c^{2} = a^{2} .$

$\begin{matrix} \sqrt{{(x - (- c))}^{2} + {(y - 0)}^{2}} + \sqrt{{(x - c)}^{2} + {(y - 0)}^{2}} & = & 2 a \\ \sqrt{{(x + c)}^{2} + y^{2}} & = & 2 a - \sqrt{{(x - c)}^{2} + y^{2}} \\ {(x + c)}^{2} + y^{2} & = & {(2 a - \sqrt{{(x - c)}^{2} + y^{2}})}^{2} \\ x^{2} + 2 c x + c^{2} + y^{2} & = & 4 a^{2} - 4 a \sqrt{{(x - c)}^{2} + y^{2}} + {(x - c)}^{2} + y^{2} \\ x^{2} + 2 c x + c^{2} + y^{2} & = & 4 a^{2} - 4 a \sqrt{{(x - c)}^{2} + y^{2}} + x^{2} - 2 c x + y^{2} \\ 2 c x & = & 4 a^{2} - 4 a \sqrt{{(x - c)}^{2} + y^{2}} - 2 c x \\ 4 c x - 4 a^{2} & = & 4 a \sqrt{{(x - c)}^{2} + y^{2}} \\ - \frac{1}{4 a} (4 c x - 4 a^{2}) & = & \sqrt{{(x - c)}^{2} + y^{2}} \\ a - \frac{c}{a} x & = & \sqrt{{(x - c)}^{2} + y^{2}} \\ a^{2} - 2 x c + \frac{c^{2}}{a^{2}} x^{2} & = & {(x - c)}^{2} + y^{2} \\ a^{2} - 2 x c + \frac{c^{2}}{a^{2}} x^{2} & = & x^{2} - 2 x c + c^{2} + y^{2} \\ a^{2} + \frac{c^{2}}{a^{2}} x^{2} & = & x^{2} + c^{2} + y^{2} \\ a^{2} + \frac{c^{2}}{a^{2}} x^{2} & = & x^{2} + c^{2} + y^{2} \\ a^{2} - c^{2} & = & x^{2} - \frac{c^{2}}{a^{2}} x^{2} + y^{2} \\ a^{2} - c^{2} & = & x^{2} (1 - \frac{c^{2}}{a^{2}}) + y^{2} \end{matrix}$

Let $1 = \frac{a^{2}}{a^{2}} .$

\begin{matrix} a^{2} - c^{2} & = & x^{2} (\frac{a^{2} - c^{2}}{a^{2}}) + y^{2} \\ 1 & = & \frac{x^{2}}{a^{2}} + \frac{y^{2}}{a^{2} - c^{2}} \end{matrix}

Because $b^{2} + c^{2} = a^{2},$ then $b^{2} = a^{2} - c^{2} .$

\begin{matrix} 1 & = & \frac{x^{2}}{a^{2}} + \frac{y^{2}}{a^{2} - c^{2}} \\ 1 & = & \frac{x^{2}}{a^{2}} + \frac{y^{2}}{b^{2}} \end{matrix}

Standard Form of the Hyperbola

$1 = \frac{x^{2}}{a^{2}} - \frac{y^{2}}{b^{2}}$

Derivation

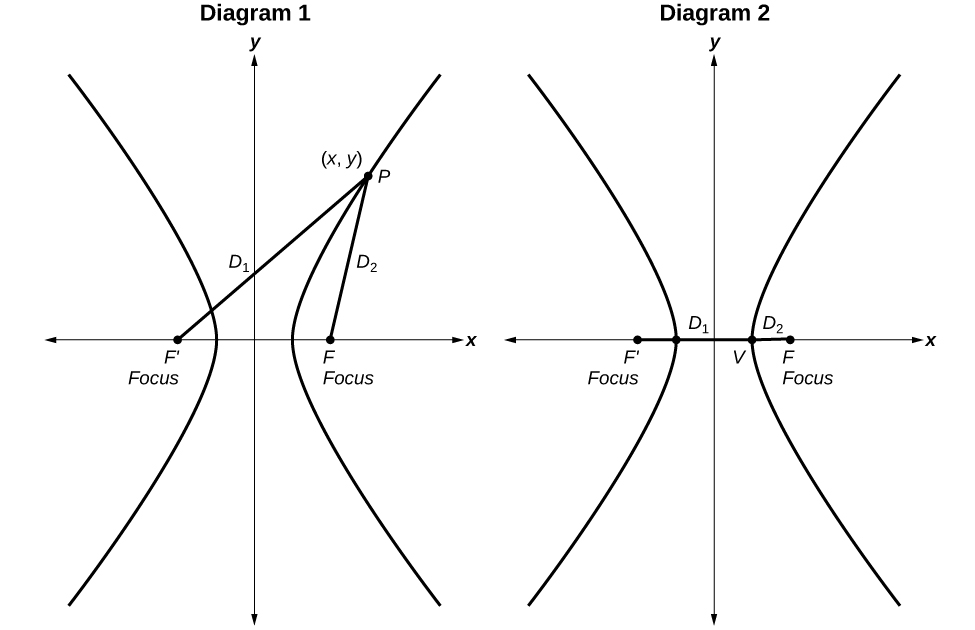
A hyperbola is the set of all points in a plane such that the absolute value of the difference of the distances between two fixed points is constant.

Side-by-side graphs of hyperbole. In Diagram 1: The foci F’ and F are labeled and can be found a little in front of the opening of the hyperbola. A point P at (x,y) on the right curve is labeled. A line extends from the F’ focus to the point P labeled: D1. A line extends from the F focus to the point P labeled: D2. In Diagram 2: The foci F’ and F are labeled and can be found a little in front of the opening of the hyperbola. A point V is labeled at the vertex of the right hyperbola. A line extends from the F’ focus to the point V labeled: D1. A line extends from the F focus to the point V labeled: D2.

Diagram 1: The difference of the distances from Point P to the foci is constant:

$\sqrt{{(x - (- c))}^{2} + {(y - 0)}^{2}} - \sqrt{{(x - c)}^{2} + {(y - 0)}^{2}} = constant$

Diagram 2: When the point is a vertex, the difference is $2 a .$

$\sqrt{{(x - (- c))}^{2} + {(y - 0)}^{2}} - \sqrt{{(x - c)}^{2} + {(y - 0)}^{2}} = 2 a$

$\begin{matrix} \sqrt{{(x - (- c))}^{2} + {(y - 0)}^{2}} - \sqrt{{(x - c)}^{2} + {(y - 0)}^{2}} & = & 2 a \\ \sqrt{{(x + c)}^{2} + y^{2}} - \sqrt{{(x - c)}^{2} + y^{2}} & = & 2 a \\ \sqrt{{(x + c)}^{2} + y^{2}} & = & 2 a + \sqrt{{(x - c)}^{2} + y^{2}} \\ {(x + c)}^{2} + y^{2} & = & (2 a + \sqrt{{(x - c)}^{2} + y^{2}}) \\ x^{2} + 2 c x + c^{2} + y^{2} & = & 4 a^{2} + 4 a \sqrt{{(x - c)}^{2}} + y^{2} \\ x^{2} + 2 c x + c^{2} + y^{2} & = & 4 a^{2} + 4 a \sqrt{{(x - c)}^{2} + y^{2}} + x^{2} - 2 c x + y^{2} \\ 2 c x & = & 4 a^{2} + 4 a \sqrt{{(x - c)}^{2} + y^{2}} - 2 c x \\ 4 c x - 4 a^{2} & = & 4 a \sqrt{{(x - c)}^{2} + y^{2}} \\ c x - a^{2} & = & a \sqrt{{(x - c)}^{2} + y^{2}} \\ {(c x - a^{2})}^{2} & = & a^{2} ({(x - c)}^{2} + y^{2}) \\ c^{2} x^{2} - 2 a^{2} c^{2} x^{2} + a^{4} & = & a^{2} x^{2} - 2 a^{2} c^{2} x^{2} + a^{2} c^{2} + a^{2} y^{2} \\ c^{2} x^{2} + a^{4} & = & a^{2} x^{2} + a^{2} c^{2} + a^{2} y^{2} \\ a^{4} - a^{2} c^{2} & = & a^{2} x^{2} - c^{2} x^{2} + a^{2} y^{2} \\ a^{2} (a^{2} - c^{2}) & = & (a^{2} - c^{2}) x^{2} + a^{2} y^{2} \\ a^{2} (a^{2} - c^{2}) & = & (c^{2} - a^{2}) x^{2} - a^{2} y^{2} \end{matrix}$

Define $b$ as a positive number such that $b^{2} = c^{2} - a^{2} .$

\begin{matrix} a^{2} b^{2} & = & b^{2} x^{2} - a^{2} y^{2} \\ \frac{a^{2} b^{2}}{a^{2} b^{2}} & = & \frac{b^{2} x^{2}}{a^{2} b^{2}} - \frac{a^{2} y^{2}}{a^{2} b^{2}} \\ 1 & = & \frac{x^{2}}{a^{2}} - \frac{y^{2}}{b^{2}} \end{matrix}

Trigonometric identities

Pythagorean Identity	$\begin{array}{l} \cos^{2} t + \sin^{2} t = 1 \\ 1 + \tan^{2} t = \sec^{2} t \\ 1 + \cot^{2} t = \csc^{2} t \end{array}$
Even-Odd Identities	$\begin{array}{l} \cos (- t) = c o s t \\ \sec (- t) = \sec t \\ \sin (- t) = - \sin t \\ \tan (- t) = - \tan t \\ \csc (- t) = - \csc t \\ \cot (- t) = - \cot t \end{array}$
Cofunction Identities	$\begin{array}{l} \cos t = \sin (\frac{π}{2} - t) \\ \sin t = \cos (\frac{π}{2} - t) \\ \tan t = \cot (\frac{π}{2} - t) \\ \cot t = \tan (\frac{π}{2} - t) \\ \sec t = \csc (\frac{π}{2} - t) \\ \csc t = \sec (\frac{π}{2} - t) \end{array}$
Fundamental Identities	$\begin{array}{l} \tan t = \frac{\sin t}{\cos t} \\ \sec t = \frac{1}{\cos t} \\ \csc t = \frac{1}{\sin t} \\ c o t t = \frac{1}{tan t} = \frac{cos t}{sin t} \end{array}$
Sum and Difference Identities	$\begin{array}{l} \cos (α + β) = \cos α \cos β - \sin α \sin β \\ \cos (α - β) = \cos α \cos β + \sin α \sin β \\ \sin (α + β) = \sin α \cos β + \cos α \sin β \\ \sin (α - β) = \sin α \cos β - \cos α \sin β \\ \tan (α + β) = \frac{\tan α + \tan β}{1 - \tan α \tan β} \\ \tan (α - β) = \frac{\tan α - \tan β}{1 + \tan α \tan β} \end{array}$
Double-Angle Formulas	$\begin{array}{l} \sin (2 θ) = 2 \sin θ \cos θ \\ \cos (2 θ) = \cos^{2} θ - \sin^{2} θ \\ \cos (2 θ) = 1 - 2 \sin^{2} θ \\ \cos (2 θ) = 2 \cos^{2} θ - 1 \\ \tan (2 θ) = \frac{2 \tan θ}{1 - \tan^{2} θ} \end{array}$
Half-Angle Formulas	$\begin{array}{l} \sin \frac{α}{2} = \pm \sqrt{\frac{1 - \cos α}{2}} \\ \cos \frac{α}{2} = \pm \sqrt{\frac{1 + \cos α}{2}} \\ \tan \frac{α}{2} = \pm \sqrt{\frac{1 - \cos α}{1 + \cos α}} \\ \tan \frac{α}{2} = \frac{\sin α}{1 + \cos α} \\ \tan \frac{α}{2} = \frac{1 - \cos α}{\sin α} \end{array}$
Reduction Formulas	$\begin{array}{l} \sin^{2} θ = \frac{1 - \cos (2 θ)}{2} \\ \cos^{2} θ = \frac{1 + \cos (2 θ)}{2} \\ \tan^{2} θ = \frac{1 - \cos (2 θ)}{1 + \cos (2 θ)} \end{array}$
Product-to-Sum Formulas	$\begin{array}{l} \cos α \cos β = \frac{1}{2} [\cos (α - β) + \cos (α + β)] \\ \sin α \cos β = \frac{1}{2} [\sin (α + β) + \sin (α - β)] \\ \sin α \sin β = \frac{1}{2} [\cos (α - β) - \cos (α + β)] \\ \cos α \sin β = \frac{1}{2} [\sin (α + β) - \sin (α - β)] \end{array}$
Sum-to-Product Formulas	$\begin{array}{l} \sin α + \sin β = 2 \sin (\frac{α + β}{2}) \cos (\frac{α - β}{2}) \\ \sin α - \sin β = 2 \sin (\frac{α - β}{2}) \cos (\frac{α + β}{2}) \\ \cos α - \cos β = - 2 \sin (\frac{α + β}{2}) \sin (\frac{α - β}{2}) \\ \cos α + \cos β = 2 \cos (\frac{α + β}{2}) \cos (\frac{α - β}{2}) \end{array}$
Law of Sines	$\begin{array}{l} \frac{\sin α}{a} = \frac{\sin β}{b} = \frac{\sin γ}{c} \\ \frac{a}{\sin α} = \frac{b}{\sin β} = \frac{c}{\sin γ} \end{array}$
Law of Cosines	$\begin{matrix} a^{2} = b^{2} + c^{2} - 2 b c \cos α \\ b^{2} = a^{2} + c^{2} - 2 a c \cos β \\ c^{2} = a^{2} + b^{2} - 2 a b cos γ \end{matrix}$

Toolkit functions

Three graphs side-by-side. From left to right, graph of the identify function, square function, and square root function. All three graphs extend from -4 to 4 on each axis.

Three graphs side-by-side. From left to right, graph of the cubic function, cube root function, and reciprocal function. All three graphs extend from -4 to 4 on each axis.

Three graphs side-by-side. From left to right, graph of the absolute value function, exponential function, and natural logarithm function. All three graphs extend from -4 to 4 on each axis.

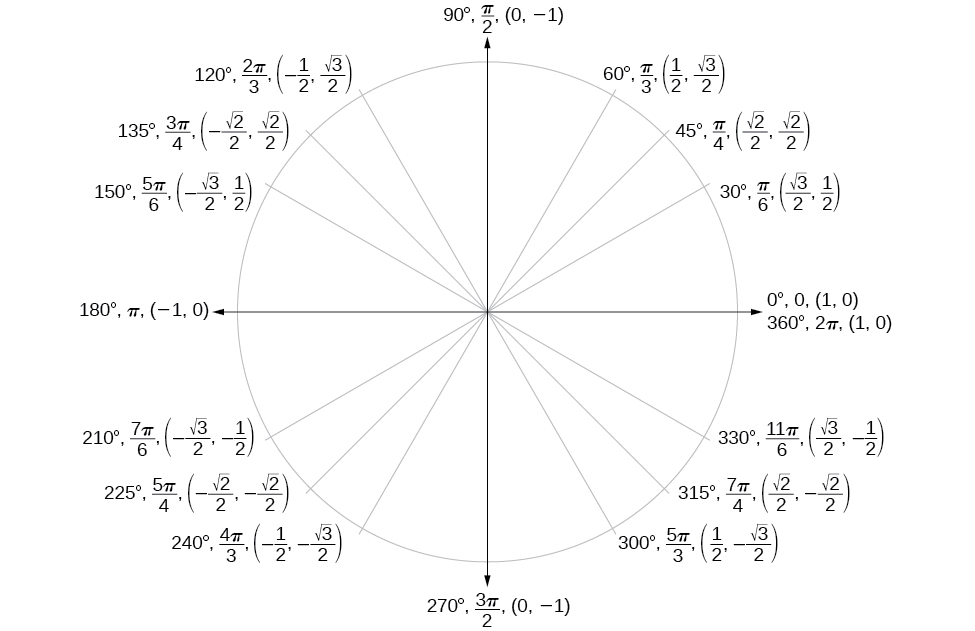
Trigonometric functions

Unit Circle

Angle	$0$	$\frac{π}{6}, or 30 °$	$\frac{π}{4}, or 45 °$	$\frac{π}{3}, or 60 °$	$\frac{π}{2}, or 90 °$
Cosine	1	$\frac{\sqrt{3}}{2}$	$\frac{\sqrt{2}}{2}$	$\frac{1}{2}$	0
Sine	0	$\frac{1}{2}$	$\frac{\sqrt{2}}{2}$	$\frac{\sqrt{3}}{2}$	1
Tangent	0	$\frac{\sqrt{3}}{3}$	1	$\sqrt{3}$	Undefined
Secant	1	$\frac{2 \sqrt{3}}{3}$	$\sqrt{2}$	2	Undefined
Cosecant	Undefined	2	$\sqrt{2}$	$\frac{2 \sqrt{3}}{3}$	1
Cotangent	Undefined	$\sqrt{3}$	1	$\frac{\sqrt{3}}{3}$	0

Questions & Answers

what is biology

Hajah Reply

the study of living organisms and their interactions with one another and their environments

AI-Robot

what is biology

Victoria Reply

HOW CAN MAN ORGAN FUNCTION

Alfred Reply

the diagram of the digestive system

Assiatu Reply

allimentary cannel

Ogenrwot

How does twins formed

William Reply

They formed in two ways first when one sperm and one egg are splited by mitosis or two sperm and two eggs join together

Oluwatobi

what is genetics

Josephine Reply

Genetics is the study of heredity

Misack

how does twins formed?

Misack

What is manual

Hassan Reply

discuss biological phenomenon and provide pieces of evidence to show that it was responsible for the formation of eukaryotic organelles

Joseph Reply

what is biology

Yousuf Reply

the study of living organisms and their interactions with one another and their environment.

Wine

discuss the biological phenomenon and provide pieces of evidence to show that it was responsible for the formation of eukaryotic organelles in an essay form

Joseph Reply

what is the blood cells

Shaker Reply

list any five characteristics of the blood cells

Shaker

lack electricity and its more savely than electronic microscope because its naturally by using of light

Abdullahi Reply

advantage of electronic microscope is easily and clearly while disadvantage is dangerous because its electronic. advantage of light microscope is savely and naturally by sun while disadvantage is not easily,means its not sharp and not clear

Abdullahi

cell theory state that every organisms composed of one or more cell,cell is the basic unit of life

Abdullahi

is like gone fail us

DENG

cells is the basic structure and functions of all living things

Ramadan

What is classification

ISCONT Reply

is organisms that are similar into groups called tara

Yamosa

in what situation (s) would be the use of a scanning electron microscope be ideal and why?

Kenna Reply

A scanning electron microscope (SEM) is ideal for situations requiring high-resolution imaging of surfaces. It is commonly used in materials science, biology, and geology to examine the topography and composition of samples at a nanoscale level. SEM is particularly useful for studying fine details,

Hilary

cell is the building block of life.

Condoleezza Reply

Got questions? Join the online conversation and get instant answers!

Jobilize.com Reply

<< Chapter < Page Page > Chapter >>

Read also:

Get Jobilize Job Search Mobile App in your pocket Now!

100% Free Mobile Applications
Receive real-time job alerts and never miss the right job again

Source: OpenStax, Algebra and trigonometry. OpenStax CNX. Nov 14, 2016 Download for free at https://legacy.cnx.org/content/col11758/1.6

Google Play and the Google Play logo are trademarks of Google Inc.

Notification Switch

Would you like to follow the 'Algebra and trigonometry' conversation and receive update notifications?

Ask

	4 Toolkit functions By OpenStax Read Online Course
	2 Important proofs and derivations By OpenStax Read Online Course
	Power Enigeering types of bearing lubrication By Sam Luong Start Quiz
	28 Biology 28 Invertebrates MCQ By OpenStax Start Quiz
	10 Physiotherapy Modalities-Thermo By Rhodes Start Quiz
	1 Human A&P 2- Test 1 By Madison Christian Start Flashcards
©flickr:	Microbiology Final By Szilárd Jankó Start Quiz
©flickr: Luis	Atoms By Carly Allen Start Quiz
	21 AP 21 Lymphatic Immune System MCQ By OpenStax Start Quiz
©flickr: Gareth	Professional Etiquette MCQ By Abby Sharp Start Quiz
	English Vocabulary By Jordon Humphreys Start Quiz
©flickr: brett	Celine Dion Quiz By JavaChamp Team Start Quiz