| << Chapter < Page | Chapter >> Page > |
Consider a block attached to a spring on a frictionless table ( [link] ). The equilibrium position (the position where the spring is neither stretched nor compressed) is marked as . At the equilibrium position, the net force is zero.
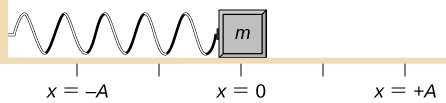
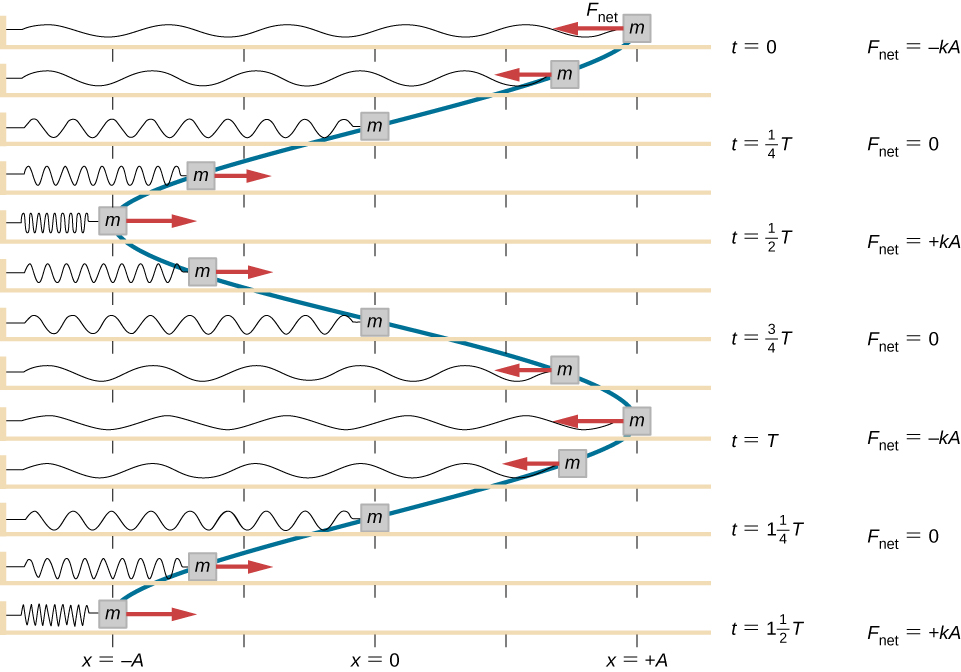
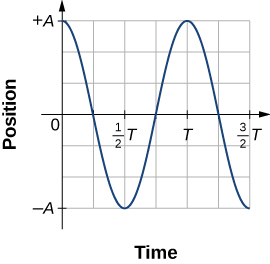
Work is done on the block to pull it out to a position of and it is then released from rest. The maximum x -position ( A ) is called the amplitude of the motion. The block begins to oscillate in SHM between and where A is the amplitude of the motion and T is the period of the oscillation. The period is the time for one oscillation. [link] shows the motion of the block as it completes one and a half oscillations after release. [link] shows a plot of the position of the block versus time. When the position is plotted versus time, it is clear that the data can be modeled by a cosine function with an amplitude A and a period T . The cosine function repeats every multiple of whereas the motion of the block repeats every period T . However, the function repeats every integer multiple of the period. The maximum of the cosine function is one, so it is necessary to multiply the cosine function by the amplitude A .
Recall from the chapter on rotation that the angular frequency equals . In this case, the period is constant, so the angular frequency is defined as divided by the period, .
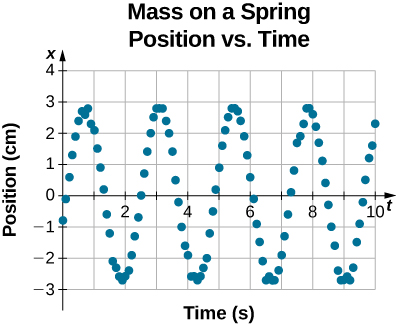
The equation for the position as a function of time is good for modeling data, where the position of the block at the initial time is at the amplitude A and the initial velocity is zero. Often when taking experimental data, the position of the mass at the initial time is not equal to the amplitude and the initial velocity is not zero. Consider 10 seconds of data collected by a student in lab, shown in [link] .

Notification Switch
Would you like to follow the 'University physics volume 1' conversation and receive update notifications?