| << Chapter < Page | Chapter >> Page > |
When we know the scalar components and of a vector , we can find its magnitude A and its direction angle . The direction angle —or direction, for short—is the angle the vector forms with the positive direction on the x -axis. The angle is measured in the counterclockwise direction from the + x -axis to the vector ( [link] ). Because the lengths A , , and form a right triangle, they are related by the Pythagorean theorem:
This equation works even if the scalar components of a vector are negative. The direction angle of a vector is defined via the tangent function of angle in the triangle shown in [link] :

When the vector lies either in the first quadrant or in the fourth quadrant, where component is positive ( [link] ), the angle in [link] ) is identical to the direction angle . For vectors in the fourth quadrant, angle is negative, which means that for these vectors, direction angle is measured clockwise from the positive x -axis. Similarly, for vectors in the second quadrant, angle is negative. When the vector lies in either the second or third quadrant, where component is negative, the direction angle is ( [link] ).
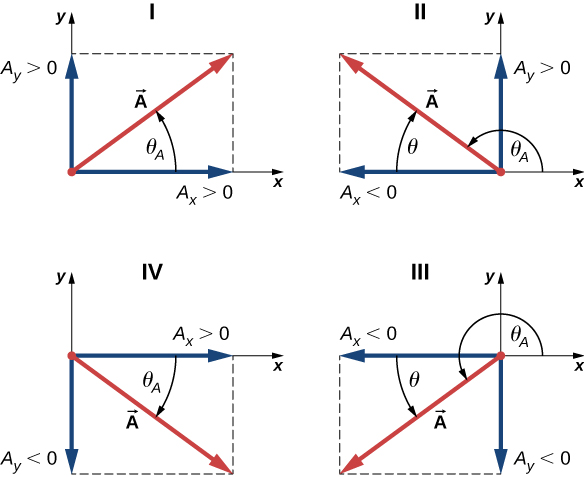
The direction angle is
Vector lies in the second quadrant, so its direction angle is
Check Your Understanding If the displacement vector of a blue fly walking on a sheet of graph paper is , find its magnitude and direction.
5.83 cm,
In many applications, the magnitudes and directions of vector quantities are known and we need to find the resultant of many vectors. For example, imagine 400 cars moving on the Golden Gate Bridge in San Francisco in a strong wind. Each car gives the bridge a different push in various directions and we would like to know how big the resultant push can possibly be. We have already gained some experience with the geometric construction of vector sums, so we know the task of finding the resultant by drawing the vectors and measuring their lengths and angles may become intractable pretty quickly, leading to huge errors. Worries like this do not appear when we use analytical methods. The very first step in an analytical approach is to find vector components when the direction and magnitude of a vector are known.

Notification Switch
Would you like to follow the 'University physics volume 1' conversation and receive update notifications?