4.4 Tangent planes and linear approximations  (Page 2/11)

Solving this equation for $z$ gives [link] .

Finding a tangent plane

Find the equation of the tangent plane to the surface defined by the function $f\left(x,y\right)=2x^2-3xy+8y^2+2x-4y+4$ at point $\left(2,\mathrm{-1}\right).$

First, we must calculate $f_x\left(x,y\right)$ and $f_y\left(x,y\right),$ then use [link] with $x_0=2$ and $y_0=\mathrm{-1}\text{:}$

$\begin{array}{ccc}\hfill f_x\left(x,y\right)& =\hfill & 4x-3y+2\hfill \\ \hfill f_y\left(x,y\right)& =\hfill & \mathrm{-3}x+16y-4\hfill \\ \hfill f\left(2,\mathrm{-1}\right)& =\hfill & 2{\left(2\right)}^2-3\left(2\right)\left(\mathrm{-1}\right)+8{\left(\mathrm{-1}\right)}^2+2\left(2\right)-4\left(\mathrm{-1}\right)+4=34.\hfill \\ \hfill f_x\left(2,\mathrm{-1}\right)& =\hfill & 4\left(2\right)-3\left(\mathrm{-1}\right)+2=13\hfill \\ \hfill f_y\left(2,\mathrm{-1}\right)& =\hfill & \mathrm{-3}\left(2\right)+16\left(\mathrm{-1}\right)-4=\mathrm{-26.}\hfill \end{array}$

Then [link] becomes

$\begin{array}{c}z=f\left(x_0,y_0\right)+f_x\left(x_0,y_0\right)\left(x-x_0\right)+f_y\left(x_0,y_0\right)\left(y-y_0\right)\hfill \\ z=34+13\left(x-2\right)-26\left(y-\left(\mathrm{-1}\right)\right)\hfill \\ z=34+13x-26-26y-26\hfill \\ z=13x-26y-18.\hfill \end{array}$

(See the following figure).

Got questions? Get instant answers now!

Got questions? Get instant answers now!

A tangent plane to a surface does not always exist at every point on the surface. Consider the function

The graph of this function follows.

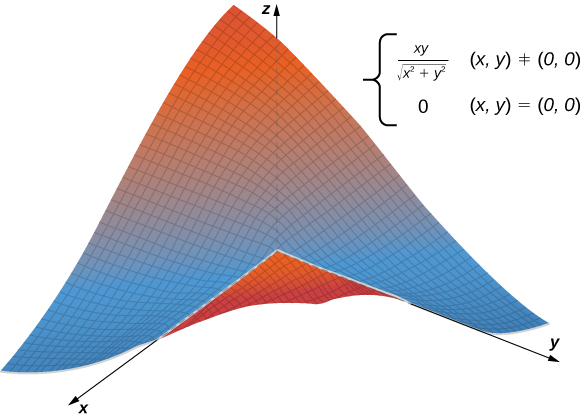
If either $x=0$ or $y=0,$ then $f\left(x,y\right)=0,$ so the value of the function does not change on either the x - or y -axis. Therefore, $f_x\left(x,0\right)=f_y\left(0,y\right)=0,$ so as either $xory$ approach zero, these partial derivatives stay equal to zero. Substituting them into [link] gives $z=0$ as the equation of the tangent line. However, if we approach the origin from a different direction, we get a different story. For example, suppose we approach the origin along the line $y=x.$ If we put $y=x$ into the original function, it becomes

When $x>0,$ the slope of this curve is equal to $\sqrt{2}\text{/}2;$ when $x<0,$ the slope of this curve is equal to $\text{−}\left(\sqrt{2}\text{/}2\right).$ This presents a problem. In the definition of tangent plane , we presumed that all tangent lines through point $P$ (in this case, the origin) lay in the same plane. This is clearly not the case here. When we study differentiable functions, we will see that this function is not differentiable at the origin.

Linear approximations

Recall from Linear Approximations and Differentials that the formula for the linear approximation of a function $f\left(x\right)$ at the point $x=a$ is given by

The diagram for the linear approximation of a function of one variable appears in the following graph.

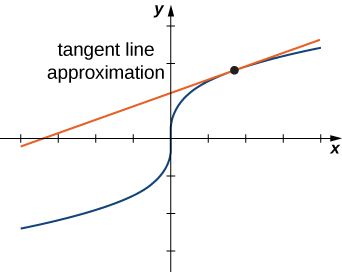
The tangent line can be used as an approximation to the function $f\left(x\right)$ for values of $x$ reasonably close to $x=a.$ When working with a function of two variables, the tangent line is replaced by a tangent plane, but the approximation idea is much the same.