2.2 Vectors in three dimensions  (Page 2/14)

Locating points in space

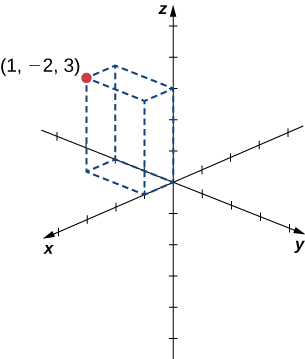
Sketch the point $\left(1,\mathrm{-2},3\right)$ in three-dimensional space.

To sketch a point, start by sketching three sides of a rectangular prism along the coordinate axes: one unit in the positive $x$ direction, $2$ units in the negative $y$ direction, and $3$ units in the positive $z$ direction. Complete the prism to plot the point ( [link] ).

Got questions? Get instant answers now!

Got questions? Get instant answers now!

In two-dimensional space, the coordinate plane is defined by a pair of perpendicular axes. These axes allow us to name any location within the plane. In three dimensions, we define coordinate planes by the coordinate axes, just as in two dimensions. There are three axes now, so there are three intersecting pairs of axes. Each pair of axes forms a coordinate plane: the xy -plane, the xz -plane, and the yz -plane ( [link] ). We define the xy -plane formally as the following set: $\left\{\left(x,y,0\right):x,y\in ℝ\right\}.$ Similarly, the xz -plane and the yz -plane are defined as $\left\{\left(x,0,z\right):x,z\in ℝ\right\}$ and $\left\{\left(0,y,z\right):y,z\in ℝ\right\},$ respectively.

To visualize this, imagine you’re building a house and are standing in a room with only two of the four walls finished. (Assume the two finished walls are adjacent to each other.) If you stand with your back to the corner where the two finished walls meet, facing out into the room, the floor is the xy -plane, the wall to your right is the xz -plane, and the wall to your left is the yz -plane.

In two dimensions, the coordinate axes partition the plane into four quadrants. Similarly, the coordinate planes divide space between them into eight regions about the origin, called octants    . The octants fill ${ℝ}^3$ in the same way that quadrants fill ${ℝ}^2,$ as shown in [link] .

Most work in three-dimensional space is a comfortable extension of the corresponding concepts in two dimensions. In this section, we use our knowledge of circles to describe spheres, then we expand our understanding of vectors to three dimensions. To accomplish these goals, we begin by adapting the distance formula to three-dimensional space.

If two points lie in the same coordinate plane, then it is straightforward to calculate the distance between them. We that the distance $d$ between two points $\left(x_1,y_1\right)$ and $\left(x_2,y_2\right)$ in the xy -coordinate plane is given by the formula

The formula for the distance between two points in space is a natural extension of this formula.

The proof of this theorem is left as an exercise. ( Hint: First find the distance $d_1$ between the points $\left(x_1,y_1,z_1\right)$ and $\left(x_2,y_2,z_1\right)$ as shown in [link] .)

Distance in space

Find the distance between points $P_1=\left(3,\text{−}1,5\right)$ and $P_2=\left(2,1,\text{−}1\right).$

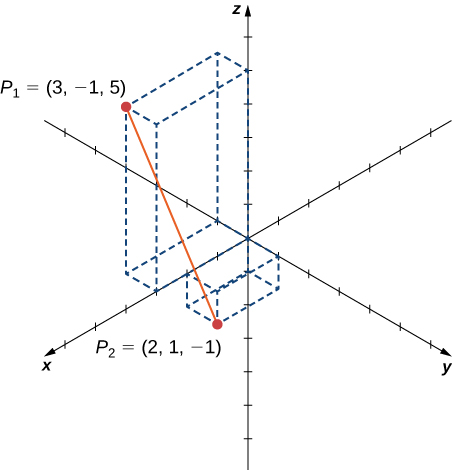
Substitute values directly into the distance formula:

$\begin{array}{cc}\hfill d\left(P_1,P_2\right)& =\sqrt{{\left(x_2-x_1\right)}^2+{\left(y_2-y_1\right)}^2+{\left(z_2-z_1\right)}^2}\hfill \\ & =\sqrt{{\left(2-3\right)}^2+{\left(1-\left(\mathrm{-1}\right)\right)}^2+{\left(\mathrm{-1}-5\right)}^2}\hfill \\ & =\sqrt{1^2+2^2+{\left(\mathrm{-6}\right)}^2}\hfill \\ & =\sqrt{41}.\hfill \end{array}$

Got questions? Get instant answers now!

Got questions? Get instant answers now!