1. Home
  2. Applied probability
  3. Independent classes of random
  4. Independent classes of random

Independent classes of random variables  (Page 2/6)

<< Chapter < Page
  Applied probability     Page 2 / 6
Chapter >> Page >

The joint mass distribution

It should be apparent that the independence condition puts restrictions on the character of the joint mass distribution on the plane. In order to describe this more succinctly,we employ the following terminology.

Definition

If M is a subset of the horizontal axis and N is a subset of the vertical axis, then the cartesian product M × N is the (generalized) rectangle consisting of those points ( t , u ) on the plane such that t ∈ M and u ∈ N .

Rectangle with interval sides

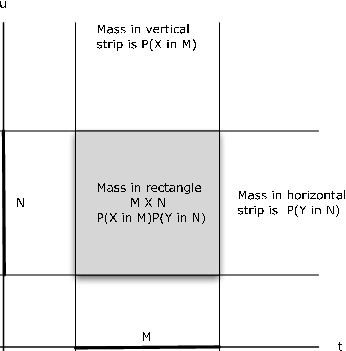
The rectangle in [link] is the Cartesian product I 1 × I 2 , consisting of all those points ( t , u ) such that a ≤ t ≤ b and c ≤ u ≤ d (i.e., t ∈ I 1 and u ∈ I 2 ).

Got questions? Get instant answers now!
A graph with two horizontal lines and two vertical lines intersecting each other and creating a square. the distance between the two vertical line is labeled M and the distance between the two horizontal lines is labeled N. The area inside the square is shaded and labeled 'Mass in rectangle MxN P(X in M)P(Y in N)'. The space above the square contains the phrase 'mass in vertical strip is P(X in M)' while the area to the right of the square is labeled 'Mass in horizontal strip is P(Y in N)'. A graph with two horizontal lines and two vertical lines intersecting each other and creating a square. the distance between the two vertical line is labeled M and the distance between the two horizontal lines is labeled N. The area inside the square is shaded and labeled 'Mass in rectangle MxN P(X in M)P(Y in N)'. The space above the square contains the phrase 'mass in vertical strip is P(X in M)' while the area to the right of the square is labeled 'Mass in horizontal strip is P(Y in N)'.
Joint distribution for an independent pair of random variables.

We restate the product rule for independence in terms of cartesian product sets.

P ( X ∈ M , Y ∈ N ) = P ( X , Y ) ∈ M × N = P ( X ∈ M ) P ( Y ∈ N )

Reference to [link] illustrates the basic pattern. If M , N are intervals on the horizontal and vertical axes, respectively, then the rectangle M × N is the intersection of the vertical strip meeting the horizontal axis in M with the horizontal strip meeting the vertical axis in N . The probability X ∈ M is the portion of the joint probability mass in the vertical strip; the probability Y ∈ N is the part of the joint probability in the horizontal strip. The probability in the rectangle is the product of these marginal probabilities.

This suggests a useful test for nonindependence which we call the rectangle test . We illustrate with a simple example.

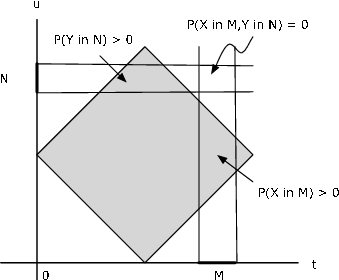
A graph containing a square rotated on one of its corners making it look like a diamond. This diamond is shaded. There are two rectangles, one rising from the x axis and another from the y axis. These two rectangles intersect different corners of the shaded diamond and each other. The area of intersection of the rectangle originating on the y axis and the diamond is labeled P(Y in N > 0). The intersection of the two rectangles is labeled P(X in M,Y in N) = 0. The area of intersection of the rectangle originating from the x-axis and the shaded diamond is labeled P(X in M) > 0. The height of the rectangle on the y axis is labeled N, and the width of the rectangle on the x axis is labeled M. A graph containing a square rotated on one of its corners making it look like a diamond. This diamond is shaded. There are two rectangles, one rising from the x axis and another from the y axis. These two rectangles intersect different corners of the shaded diamond and each other. The area of intersection of the rectangle originating on the y axis and the diamond is labeled P(Y in N > 0). The intersection of the two rectangles is labeled P(X in M,Y in N) = 0. The area of intersection of the rectangle originating from the x-axis and the shaded diamond is labeled P(X in M) > 0. The height of the rectangle on the y axis is labeled N, and the width of the rectangle on the x axis is labeled M.
Rectangle test for nonindependence of a pair of random variables.

The rectangle test for nonindependence

Supose probability mass is uniformly distributed over the square with vertices at (1,0), (2,1), (1,2), (0,1). It is evident from [link] that a value of X determines the possible values of Y and vice versa, so that we would not expect independence of the pair. To establish this, consider the small rectangle M × N shown on the figure. There is no probability mass in the region. Yet P ( X ∈ M ) > 0 and P ( Y ∈ N ) > 0 , so that

P ( X ∈ M ) P ( Y ∈ N ) > 0 , but P ( X , Y ) ∈ M × N = 0 . The product rule fails; hence the pair cannot be stochastically independent.

Got questions? Get instant answers now!

Remark . There are nonindependent cases for which this test does not work. And it does not provide a test for independence. In spite of these limitations, it is frequently useful.Because of the information contained in the independence condition, in many cases the complete joint and marginal distributions may be obtained with appropriate partialinformation. The following is a simple example.

Joint and marginal probabilities from partial information

Suppose the pair { X , Y } is independent and each has three possible values. The following four items of information are available.

P ( X = t 1 ) = 0 . 2 , P ( Y = u 1 ) = 0 . 3 , P ( X = t 1 , Y = u 2 ) = 0 . 08
P ( X = t 2 , Y = u 1 ) = 0 . 15

These values are shown in bold type on [link] . A combination of the product rule and the fact that the total probability mass is one are used to calculate each ofthe marginal and joint probabilities. For example P ( X = t 1 ) = 0 . 2 and P ( X = t 1 , Y = u 2 )

= P ( X = t 1 ) P ( Y = u 2 ) = 0 . 08 implies P ( Y = u 2 ) = 0 . 4 . Then P ( Y = u 3 )

= 1 - P ( Y = u 1 ) - P ( Y = u 2 ) = 0 . 3 . Others are calculated similarly. There is no unique procedure for solution. And it has not seemeduseful to develop MATLAB procedures to accomplish this.

Got questions? Get instant answers now!
<< Chapter < Page Page > Chapter >>

Read also:

Get Jobilize Job Search Mobile App in your pocket Now!

Get it on Google Play Download on the App Store Now




Source:  OpenStax, Applied probability. OpenStax CNX. Aug 31, 2009 Download for free at http://cnx.org/content/col10708/1.6
Google Play and the Google Play logo are trademarks of Google Inc.

Notification Switch

Would you like to follow the 'Applied probability' conversation and receive update notifications?

Ask