5.6 Calculating centers of mass and moments of inertia (Page 4/8)

Calculus volume 3 Page 4 / 8

R_{x} = \sqrt{\frac{I_{x}}{m}}, R_{y} = \sqrt{\frac{I_{y}}{m}}, and R_{0} = \sqrt{\frac{I_{0}}{m}},

respectively. In each case, the radius of gyration tells us how far (perpendicular distance) from the axis of rotation the entire mass of an object might be concentrated. The moments of an object are useful for finding information on the balance and torque of the object about an axis, but radii of gyration are used to describe the distribution of mass around its centroidal axis. There are many applications in engineering and physics. Sometimes it is necessary to find the radius of gyration, as in the next example.

Finding the radius of gyration for a triangular lamina

Consider the same triangular lamina $R$ with vertices $(0, 0), (2, 2),$ and $(2, 0)$ and with density $ρ (x, y) = x y$ as in previous examples. Find the radii of gyration with respect to the $x -axis,$ the $y -axis,$ and the origin.

If we compute the mass of this region we find that $m = 2 .$ We found the moments of inertia of this lamina in [link] . From these data, the radii of gyration with respect to the $x -axis,$ $y -axis,$ and the origin are, respectively,

\begin{matrix} R_{x} & = & \sqrt{\frac{I_{x}}{m}} = \sqrt{\frac{8 / 3}{2}} = \sqrt{\frac{8}{6}} = \frac{2 \sqrt{3}}{3}, \\ R_{y} & = & \sqrt{\frac{I_{y}}{m}} = \sqrt{\frac{16 / 3}{2}} = \sqrt{\frac{8}{3}} = \frac{2 \sqrt{6}}{3}, \\ R_{0} & = & \sqrt{\frac{I_{0}}{m}} = \sqrt{\frac{8}{2}} = \sqrt{4} = 2 . \end{matrix}

Got questions? Get instant answers now!

Use the same region $R$ from [link] and the density function $ρ (x, y) = \sqrt{x y} .$ Find the radii of gyration with respect to the $x -axis,$ the $y -axis,$ and the origin.

$R_{x} = \frac{6 \sqrt{35}}{35},$ $R_{y} = \frac{6 \sqrt{15}}{15},$ and $R_{0} = \frac{4 \sqrt{42}}{7} .$

Got questions? Get instant answers now!

Center of mass and moments of inertia in three dimensions

All the expressions of double integrals discussed so far can be modified to become triple integrals.

Definition

If we have a solid object $Q$ with a density function $ρ (x, y, z)$ at any point $(x, y, z)$ in space, then its mass is

m = \underset{Q}{∭} ρ (x, y, z) d V .

Its moments about the $x y -plane,$ the $x z -plane,$ and the $y z -plane$ are

\begin{matrix} M_{x y} = \underset{Q}{∭} z ρ (x, y, z) d V, M_{x z} = \underset{Q}{∭} y ρ (x, y, z) d V, \\ M_{y z} = \underset{Q}{∭} x ρ (x, y, z) d V . \end{matrix}

If the center of mass of the object is the point $(\overset{−}{x}, \overset{−}{y}, \overset{−}{z}),$ then

\overset{−}{x} = \frac{M_{y z}}{m}, \overset{−}{y} = \frac{M_{x z}}{m}, \overset{−}{z} = \frac{M_{x y}}{m} .

Also, if the solid object is homogeneous (with constant density), then the center of mass becomes the centroid of the solid. Finally, the moments of inertia about the $y z -plane,$ the $x z -plane,$ and the $x y -plane$ are

\begin{matrix} I_{x} = \underset{Q}{∭} (y^{2} + z^{2}) ρ (x, y, z) d V, \\ I_{y} = \underset{Q}{∭} (x^{2} + z^{2}) ρ (x, y, z) d V, \\ I_{z} = \underset{Q}{∭} (x^{2} + y^{2}) ρ (x, y, z) d V . \end{matrix}

Finding the mass of a solid

Suppose that $Q$ is a solid region bounded by $x + 2 y + 3 z = 6$ and the coordinate planes and has density $ρ (x, y, z) = x^{2} y z .$ Find the total mass.

The region $Q$ is a tetrahedron ( [link] ) meeting the axes at the points $(6, 0, 0), (0, 3, 0),$ and $(0, 0, 2) .$ To find the limits of integration, let $z = 0$ in the slanted plane $z = \frac{1}{3} (6 - x - 2 y) .$ Then for $x$ and $y$ find the projection of $Q$ onto the $x y -plane,$ which is bounded by the axes and the line $x + 2 y = 6 .$ Hence the mass is

m = \underset{Q}{∭} ρ (x, y, z) d V = \int_{x = 0}^{x = 6} \int_{y = 0}^{y = 1 / 2 (6 - x)} \int_{z = 0}^{z = 1 / 3 (6 - x - 2 y)} x^{2} y z d z d y d x = \frac{108}{35} \approx 3.086 .

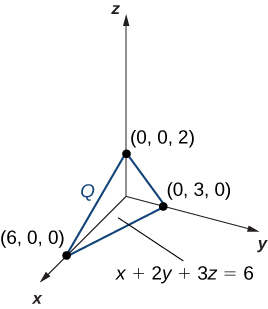
In x y z space, the solid Q is shown with corners (0, 0, 0), (0, 0, 2), (0, 3, 0), and (6, 0, 0). Alternatively, you could consider the solid as being bounded by the x y, x z, and y z planes and the plane x + 2y + 3z = 6, forming an irregular tetrahedron. — Finding the mass of a three-dimensional solid $Q .$

Got questions? Get instant answers now!

Consider the same region $Q$ ( [link] ), and use the density function $ρ (x, y, z) = x y^{2} z .$ Find the mass.

$\frac{54}{35} = 1.543$

Got questions? Get instant answers now!

Finding the center of mass of a solid

Suppose $Q$ is a solid region bounded by the plane $x + 2 y + 3 z = 6$ and the coordinate planes with density $ρ (x, y, z) = x^{2} y z$ (see [link] ). Find the center of mass using decimal approximation.

We have used this tetrahedron before and know the limits of integration, so we can proceed to the computations right away. First, we need to find the moments about the $x y -plane,$ the $x z -plane,$ and the $y z -plane:$

\begin{matrix} M_{x y} = \underset{Q}{∭} z ρ (x, y, z) d V = \int_{x = 0}^{x = 6} \int_{y = 0}^{y = 1 / 2 (6 - x)} \int_{z = 0}^{z = 1 / 3 (6 - x - 2 y)} x^{2} y z^{2} d z d y d x = \frac{54}{35} \approx 1.543, \\ M_{x z} = \underset{Q}{∭} y ρ (x, y, z) d V = \int_{x = 0}^{x = 6} \int_{y = 0}^{y = 1 / 2 (6 - x)} \int_{z = 0}^{z = 1 / 3 (6 - x - 2 y)} x^{2} y^{2} z d z d y d x = \frac{81}{35} \approx 2.314, \\ M_{y z} = \underset{Q}{∭} x ρ (x, y, z) d V = \int_{x = 0}^{x = 6} \int_{y = 0}^{y = 1 / 2 (6 - x)} \int_{z = 0}^{z = 1 / 3 (6 - x - 2 y)} x^{3} y z d z d y d x = \frac{243}{35} \approx 6.943 . \end{matrix}

Hence the center of mass is

\begin{matrix} \overset{−}{x} = \frac{M_{y z}}{m}, \overset{−}{y} = \frac{M_{x z}}{m}, \overset{−}{z} = \frac{M_{x y}}{m}, \\ \overset{−}{x} = \frac{M_{y z}}{m} = \frac{243 / 35}{108 / 35} = \frac{243}{108} = 2.25, \\ \overset{−}{y} = \frac{M_{x z}}{m} = \frac{81 / 35}{108 / 35} = \frac{81}{108} = 0.75, \\ \overset{−}{z} = \frac{M_{x y}}{m} = \frac{54 / 35}{108 / 35} = \frac{54}{108} = 0.5 . \end{matrix}

The center of mass for the tetrahedron $Q$ is the point $(2.25, 0.75, 0.5) .$

Got questions? Get instant answers now!

<< Chapter < Page Page > Chapter >>

Practice Key Terms 1

Read also:

Get Jobilize Job Search Mobile App in your pocket Now!

100% Free Mobile Applications
Receive real-time job alerts and never miss the right job again

Source: OpenStax, Calculus volume 3. OpenStax CNX. Feb 05, 2016 Download for free at http://legacy.cnx.org/content/col11966/1.2

Google Play and the Google Play logo are trademarks of Google Inc.

Notification Switch

Would you like to follow the 'Calculus volume 3' conversation and receive update notifications?

Ask

©flickr: Gisela	Electrocardiogram Quiz By Anonymous User Start Quiz
	22 AP 22 Respiratory System MCQ By OpenStax Start Quiz
©flickr: Justin	The Last Holiday Concert Chapter 1 By Mackenzie Wilcox Start Quiz
	Assembly Programming Language By JavaChamp Team Start Quiz
	NCE Ch 11 Counseling Families, Diagnosis... By Anh Dao Start Quiz
	Vocabulary for "A Rose for Emily" By Bonnie Hurst Start Quiz
	3 Pharmacology Excl. Nervous System MCQ By Rohini Ajay Start Quiz
	Computer Skills Literacy MCQ By LaToya Trowers Start Quiz
©flickr: Hey	Anatomy Physiology Final By Joli Julianna Start Test
	Business Law Ethics By Kevin Moquin Start Quiz