5.1 Sequences (Page 6/25)

Calculus volume 2 Page 6 / 25

lim_{n \to \infty} \sqrt{5 - \frac{3}{n^{2}}} = \sqrt{lim_{n \to \infty} (5 - \frac{3}{n^{2}})} = \sqrt{5} .

Continuous functions defined on convergent sequences

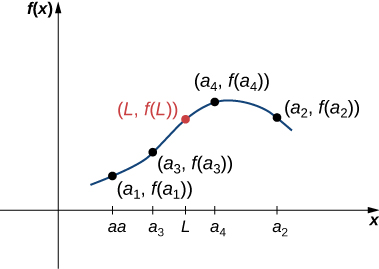
Consider a sequence ${a_{n}}$ and suppose there exists a real number $L$ such that the sequence ${a_{n}}$ converges to $L .$ Suppose $f$ is a continuous function at $L .$ Then there exists an integer $N$ such that $f$ is defined at all values $a_{n}$ for $n \geq N,$ and the sequence ${f (a_{n})}$ converges to $f (L)$ ( [link] ).

Proof

Let $ϵ > 0 .$ Since $f$ is continuous at $L,$ there exists $δ > 0$ such that $| f (x) - f (L) | < ε$ if $| x - L | < δ .$ Since the sequence ${a_{n}}$ converges to $L,$ there exists $N$ such that $| a_{n} - L | < δ$ for all $n \geq N .$ Therefore, for all $n \geq N,$ $| a_{n} - L | < δ,$ which implies $| f (a_{n}) − f (L) | < ε .$ We conclude that the sequence ${f (a_{n})}$ converges to $f (L) .$

□

A graph in quadrant 1 with points (a_1, f(a_1)), (a_3, f(a_3)), (L, f(L)), (a_4, f(a_4)), and (a_2, f(a_2)) connected by smooth curves. — Because $f$ is a continuous function as the inputs $a_{1}, a_{2}, a_{3},…$ approach $L,$ the outputs $f (a_{1}), f (a_{2}), f (a_{3}),…$ approach $f (L) .$

Limits involving continuous functions defined on convergent sequences

Determine whether the sequence ${cos (3 / n^{2})}$ converges. If it converges, find its limit.

Since the sequence ${3 / n^{2}}$ converges to $0$ and $cos x$ is continuous at $x = 0,$ we can conclude that the sequence ${cos (3 / n^{2})}$ converges and

lim_{n \to \infty} cos (\frac{3}{n^{2}}) = cos (0) = 1 .

Got questions? Get instant answers now!

Determine if the sequence ${\sqrt{\frac{2 n + 1}{3 n + 5}}}$ converges. If it converges, find its limit.

The sequence converges, and its limit is $\sqrt{2 / 3} .$

Got questions? Get instant answers now!

Another theorem involving limits of sequences is an extension of the Squeeze Theorem for limits discussed in Introduction to Limits .

Squeeze theorem for sequences

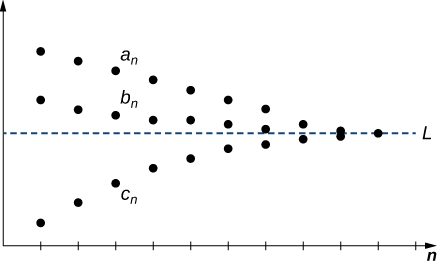
Consider sequences ${a_{n}},$ ${b_{n}},$ and ${c_{n}} .$ Suppose there exists an integer $N$ such that

a_{n} \leq b_{n} \leq c_{n} for all n \geq N .

If there exists a real number $L$ such that

lim_{n \to \infty} a_{n} = L = lim_{n \to \infty} c_{n},

then ${b_{n}}$ converges and $lim_{n \to \infty} b_{n} = L$ ( [link] ).

Proof

Let $ε > 0 .$ Since the sequence ${a_{n}}$ converges to $L,$ there exists an integer $N_{1}$ such that $| a_{n} - L | < ε$ for all $n \geq N_{1} .$ Similarly, since ${c_{n}}$ converges to $L,$ there exists an integer $N_{2}$ such that $| c_{n} - L | < ε$ for all $n \geq N_{2} .$ By assumption, there exists an integer $N$ such that $a_{n} \leq b_{n} \leq c_{n}$ for all $n \geq N .$ Let $M$ be the largest of $N_{1}, N_{2},$ and $N .$ We must show that $| b_{n} - L | < ε$ for all $n \geq M .$ For all $n \geq M,$

− ε < − | a_{n} - L | \leq a_{n} - L \leq b_{n} - L \leq c_{n} - L \leq | c_{n} - L | < ε .

Therefore, $− ε < b_{n} - L < ε,$ and we conclude that $| b_{n} - L | < ε$ for all $n \geq M,$ and we conclude that the sequence ${b_{n}}$ converges to $L .$

□

A graph in quadrant 1 with the line y = L and the x axis labeled as the n axis. Points are plotted above and below the line, converging to L as n goes to infinity. Points a_n, b_n, and c_n are plotted at the same n-value. A_n and b_n are above y = L, and c_n is below it. — Each term $b_{n}$ satisfies $a_{n} \leq b_{n} \leq c_{n}$ and the sequences ${a_{n}}$ and ${c_{n}}$ converge to the same limit, so the sequence ${b_{n}}$ must converge to the same limit as well.

Using the squeeze theorem

Use the Squeeze Theorem to find the limit of each of the following sequences.

${\frac{cos n}{n^{2}}}$
${{(- \frac{1}{2})}^{n}}$

Since $−1 \leq cos n \leq 1$ for all integers $n,$ we have

$- \frac{1}{n^{2}} \leq \frac{cos n}{n^{2}} \leq \frac{1}{n^{2}} .$

Since $−1 / n^{2} \to 0$ and $1 / n^{2} \to 0,$ we conclude that $cos n / n^{2} \to 0$ as well.
Since

$- \frac{1}{2^{n}} \leq {(- \frac{1}{2})}^{n} \leq \frac{1}{2^{n}}$

for all positive integers $n,$ $−1 / 2^{n} \to 0$ and $1 / 2^{n} \to 0,$ we can conclude that ${(−1 / 2)}^{n} \to 0 .$

Got questions? Get instant answers now!

Find $lim_{n \to \infty} \frac{2 n - sin n}{n} .$

$2$

Got questions? Get instant answers now!

Using the idea from [link] b. we conclude that $r^{n} \to 0$ for any real number $r$ such that $−1 < r < 0 .$ If $r < − 1,$ the sequence ${r^{n}}$ diverges because the terms oscillate and become arbitrarily large in magnitude. If $r = −1,$ the sequence ${r^{n}} = {{(−1)}^{n}}$ diverges, as discussed earlier. Here is a summary of the properties for geometric sequences.

r^{n} \to 0 if | r | < 1

r^{n} \to 1 if r = 1

r^{n} \to \infty if r > 1

{r^{n}} diverges if r \leq − 1

Bounded sequences

We now turn our attention to one of the most important theorems involving sequences: the Monotone Convergence Theorem. Before stating the theorem, we need to introduce some terminology and motivation. We begin by defining what it means for a sequence to be bounded.

<< Chapter < Page Page > Chapter >>

Practice Key Terms 15

Read also:

Get Jobilize Job Search Mobile App in your pocket Now!

100% Free Mobile Applications
Receive real-time job alerts and never miss the right job again

Source: OpenStax, Calculus volume 2. OpenStax CNX. Feb 05, 2016 Download for free at http://cnx.org/content/col11965/1.2

Google Play and the Google Play logo are trademarks of Google Inc.

Notification Switch

Would you like to follow the 'Calculus volume 2' conversation and receive update notifications?

Ask

©flickr: Abraham	Biology Exam 3 By Vanessa Soledad Start Exam
	Interviewing Skills MCQ By Mary Matera Start Quiz
	1 Arts Society: Theater 1 By Jonathan Long Start Quiz
©flickr:	Arctic Ease Telemarketing Campaign Quiz By Nicole Duquette Start Quiz
	35 Biology 35 The Nervous System MCQ By OpenStax Start Quiz
	Kira Kira Test By Briana Knowlton Start Quiz
	Job Search Skills MCQ By Mary Matera Start Quiz
	46 Biology 46 Ecosystems MCQ By OpenStax Start Quiz
	2 Timeshare 2 By Jams Kalo Start Quiz
	Music 113 By Eric Crawford Start Quiz