0.7 Generalizations of the basic multiresolution wavelet system  (Page 9/28)

Two channel biorthogonal filter banks

In previous chapters for orthogonal wavelets, the analysis filters and synthesis filters are time reversal of each other; i.e., $\tilde{h}\left(n\right)=h(-n)$ , $\tilde{g}\left(n\right)=g(-n)$ . Here, for the biorthogonal case, we relax these restrictions. However, in order to perfectlyreconstruct the input, these four filters still have to satisfy a set of relations.

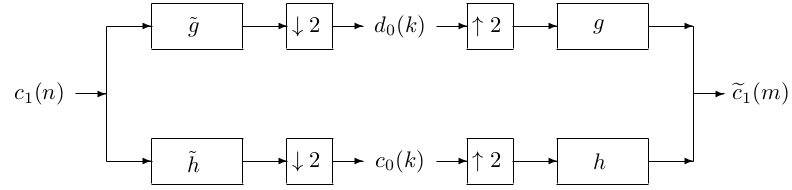
Let $c_1\left(n\right),n\in \mathbf{Z}$ be the input to the filter banks in [link] , then the outputs of the analysis filter banks are

The output of the synthesis filter bank is

Substituting Equation  [link] into [link] and interchanging the summations gives

For perfect reconstruction, i.e., ${\tilde{c}}_1\left(m\right)=c_1\left(m\right),\forall m\in \mathbf{Z}$ , we need

Fortunately, this condition can be greatly simplified. In order for it to hold, the four filters have to be related as [link]

up to some constant factors. Thus they are cross-related by time reversal and flipping signs of every other element. Clearly, when $\tilde{h}=h$ , we get the familiar relations between the scaling coefficients and the wavelet coefficients for orthogonal wavelets, $g\left(n\right)={(-1)}^{n}h(1-n)$ . Substituting [link] back to [link] , we get

In the orthogonal case, we have ${\sum }_{n}h\left(n\right)h(n+2k)=\delta \left(k\right)$ ; i.e., $h\left(n\right)$ is orthogonal to even translations of itself. Here $\tilde{h}$ is orthogonal to $h$ , thus the name biorthogonal .

Equation  [link] is the key to the understanding of the biorthogonal filter banks. Let's assume $\tilde{h}\left(n\right)$ is nonzero when ${\tilde{N}}_1\le n\le {\tilde{N}}_2$ , and $h\left(n\right)$ is nonzero when $N_1\le n\le N_2$ . Equation  [link] implies that [link]

In the orthogonal case, this reduces to the well-known fact that the length of $h$ has to be even.  [link] also imply that the difference between the lengths of $\tilde{h}$ and $h$ must be even. Thus their lengths must be both even or both odd.

Biorthogonal wavelets

We now look at the scaling function and wavelet to see how removing orthogonality and introducing a dual basis changes their characteristics.We start again with the basic multiresolution definition of the scaling function and add to that a similar definition of a dual scaling function.

From Theorem  [link] in  Chapter: The Scaling Function and Scaling Coefficients, Wavelet and Wavelet Coefficients , we know that for $\phi$ and $\tilde{\phi }$ to exist,

Continuing to parallel the construction of the orthogonal wavelets, we also define the wavelet and the dual wavelet as

Now that we have the scaling and wavelet functions and their duals, the question becomes whether we can expand and reconstruct arbitrary functionsusing them. The following theorem [link] answers this important question.

Theorem 37 For $\tilde{h}$ and $h$ satisfying [link] , suppose that for some C, $ϵ>0$ ,

If $\Phi$ and $\tilde{\Phi }$ defined above have sufficient decay in the frequency domain, then ${\psi }_{j,k}\stackrel{\mathrm{def}}{=}{2}^{j/2}\psi (2^{j}x-k),j,k\in \mathbf{Z}$ constitute a frame in $L^2\left(\mathbf{R}\right)$ . Their dual frame is given by ${\tilde{\psi }}_{j,k}\stackrel{\mathrm{def}}{=}{2}^{j/2}\tilde{\psi }(2^{j}x-k),j,k\in \mathbf{Z}$ ; for any $f\in L^2\left(\mathbf{R}\right)$ ,