4.1 Related rates  (Page 3/7)

Step 1. Draw a picture introducing the variables.

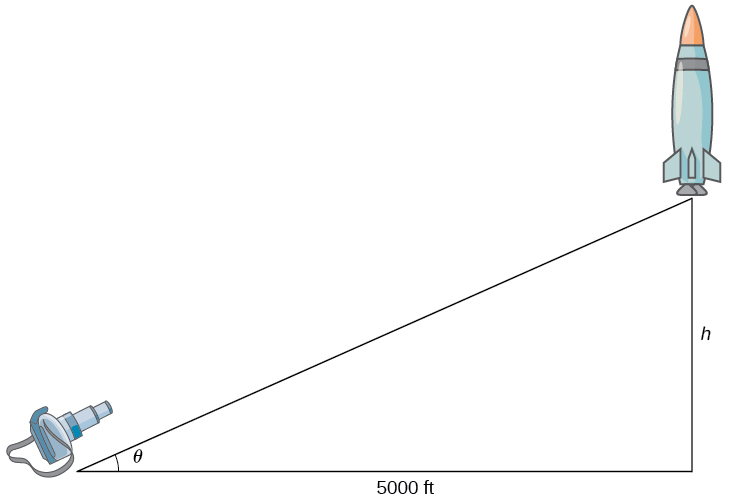
Let $h$ denote the height of the rocket above the launch pad and $\theta$ be the angle between the camera lens and the ground.

Step 2. We are trying to find the rate of change in the angle of the camera with respect to time when the rocket is 1000 ft off the ground. That is, we need to find $\frac{d\theta }{dt}$ when $h=1000\text{ft}.$ At that time, we know the velocity of the rocket is $\frac{dh}{dt}=600\text{ft/sec}.$

Step 3. Now we need to find an equation relating the two quantities that are changing with respect to time: $h$ and $\theta .$ How can we create such an equation? Using the fact that we have drawn a right triangle, it is natural to think about trigonometric functions. Recall that $\text{tan}\theta$ is the ratio of the length of the opposite side of the triangle to the length of the adjacent side. Thus, we have

This gives us the equation

Step 4. Differentiating this equation with respect to time $t,$ we obtain

Step 5. We want to find $\frac{d\theta }{dt}$ when $h=1000\text{ft}.$ At this time, we know that $\frac{dh}{dt}=600\text{ft/sec}.$ We need to determine ${\text{sec}}^2\theta .$ Recall that $\text{sec}\theta$ is the ratio of the length of the hypotenuse to the length of the adjacent side. We know the length of the adjacent side is $5000\text{ft}.$ To determine the length of the hypotenuse, we use the Pythagorean theorem, where the length of one leg is $5000\text{ft},$ the length of the other leg is $h=1000\text{ft},$ and the length of the hypotenuse is $c$ feet as shown in the following figure.

We see that

and we conclude that the hypotenuse is

Therefore, when $h=1000,$ we have

Recall from step 4 that the equation relating $\frac{d\theta }{dt}$ to our known values is

When $h=1000\text{ft},$ we know that $\frac{dh}{dt}=600\text{ft/sec}$ and ${\text{sec}}^2\theta =\frac{26}{25}.$ Substituting these values into the previous equation, we arrive at the equation

Therefore, $\frac{d\theta }{dt}=\frac{3}{26}\text{rad/sec}.$