| << Chapter < Page | Chapter >> Page > |
It is useful to point out that functions of the form where n is a positive integer, have infinite limits as x approaches a from either the left or right ( [link] ). These limits are summarized in [link] .

If n is a positive even integer, then
If n is a positive odd integer, then
and
We should also point out that in the graphs of points on the graph having x -coordinates very near to a are very close to the vertical line That is, as x approaches a , the points on the graph of are closer to the line The line is called a vertical asymptote of the graph. We formally define a vertical asymptote as follows:
Let be a function. If any of the following conditions hold, then the line is a vertical asymptote of
Evaluate each of the following limits using [link] . Identify any vertical asymptotes of the function
Evaluate each of the following limits. Identify any vertical asymptotes of the function
a. b. c. DNE. The line is the vertical asymptote of
In the next example we put our knowledge of various types of limits to use to analyze the behavior of a function at several different points.
Use the graph of in [link] to determine each of the following values:
![The graph of a function f(x) described by the above limits and values. There is a smooth curve for values below x=-2; at (-2, 3), there is an open circle. There is a smooth curve between (-2, 1] with a closed circle at (1,6). There is an open circle at (1,3), and a smooth curve stretching from there down asymptotically to negative infinity along x=3. The function also curves asymptotically along x=3 on the other side, also stretching to negative infinity. The function then changes concavity in the first quadrant around y=4.5 and continues up.](/ocw/mirror/col11964/m53491/CNX_Calc_Figure_02_02_015.jpg)
Using [link] and the graph for reference, we arrive at the following values:

In the chapter opener we mentioned briefly how Albert Einstein showed that a limit exists to how fast any object can travel. Given Einstein’s equation for the mass of a moving object, what is the value of this bound?
Our starting point is Einstein’s equation for the mass of a moving object,
where is the object’s mass at rest, v is its speed, and c is the speed of light. To see how the mass changes at high speeds, we can graph the ratio of masses as a function of the ratio of speeds, ( [link] ).
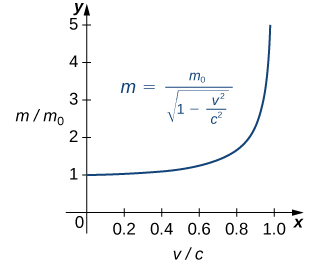
We can see that as the ratio of speeds approaches 1—that is, as the speed of the object approaches the speed of light—the ratio of masses increases without bound. In other words, the function has a vertical asymptote at We can try a few values of this ratio to test this idea.
| 0.99 | 0.1411 | 7.089 |
| 0.999 | 0.0447 | 22.37 |
| 0.9999 | 0.0141 | 70.71 |
Thus, according to [link] , if an object with mass 100 kg is traveling at 0.9999 c , its mass becomes 7071 kg. Since no object can have an infinite mass, we conclude that no object can travel at or more than the speed of light.

Notification Switch
Would you like to follow the 'Calculus volume 1' conversation and receive update notifications?