| << Chapter < Page | Chapter >> Page > |
The floor of your room is horizontal. Its slope is 0. If you carefully placed a ball on the floor, it would not roll away.
Now, we’ll consider a vertical line, the line.
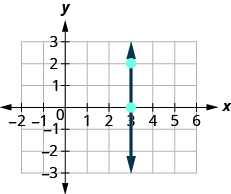
But we can’t divide by 0. Division by 0 is not defined. So we say that the slope of the vertical line is undefined.
The slope of any vertical line is undefined. When the x -coordinates of a line are all the same, the run is 0.
The slope of a vertical line, , is undefined.
Find the slope of each line:
ⓐ ⓑ .
ⓐ
This is a vertical line.
Its slope is undefined.
ⓑ
This is a horizontal line.
It has slope 0.
Remember, we ‘read’ a line from left to right, just like we read written words in English.
Sometimes we’ll need to find the slope of a line between two points when we don’t have a graph to count out the rise and the run. We could plot the points on grid paper, then count out the rise and the run, but as we’ll see, there is a way to find the slope without graphing. Before we get to it, we need to introduce some algebraic notation.
We have seen that an ordered pair gives the coordinates of a point. But when we work with slopes, we use two points. How can the same symbol be used to represent two different points? Mathematicians use subscripts to distinguish the points.
The use of subscripts in math is very much like the use of last name initials in elementary school. Maybe you remember Laura C. and Laura M. in your third grade class?
We will use to identify the first point and to identify the second point.
If we had more than two points, we could use , , and so on.
Let’s see how the rise and run relate to the coordinates of the two points by taking another look at the slope of the line between the points and .
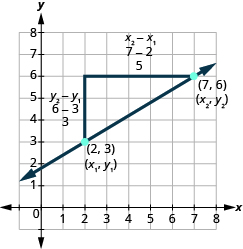
Since we have two points, we will use subscript notation, .
On the graph, we counted the rise of 3 and the run of 5.
Notice that the rise of 3 can be found by subtracting the y -coordinates 6 and 3.
And the run of 5 can be found by subtracting the x -coordinates 7 and 2.
We know . So .
We rewrite the rise and run by putting in the coordinates .
But 6 is , the y -coordinate of the second point and 3 is , the y -coordinate of the first point.
So we can rewrite the slope using subscript notation.
Also, 7 is , the x -coordinate of the second point and 2 is , the x -coordinate of the first point.
So, again, we rewrite the slope using subscript notation.
We’ve shown that is really another version of . We can use this formula to find the slope of a line when we have two points on the line.

Notification Switch
Would you like to follow the 'Elementary algebra' conversation and receive update notifications?